When producing a new adhesive or sealant product, the formulation must first be worked out. The next step, which requires a scientific yet artful eye, is mixing and dispersion to take the adhesive or sealant formulation to its end-use state. Proper care during the mixing process ensures that time and energy input is minimized while the utility of the formulation is optimized.

Understanding Silicones

Many factors make silicones unique in the world of adhesives and sealants, and polymers in general. These include their chemical structure, as well as physical properties and phenomena exhibited, such as permeability of the finished (cured) product, rheological characteristics, and their interaction with the environment surrounding them. Silicones behave differently than many other polymer adhesives and sealants. This is due to their inorganic silicon-oxygen backbone, compared to the organic carbon-hydrogen backbone of most polymers. Silicones exhibit the lowest glass transition temperature (T_g) and highest permeability of any polymer—two properties that impact the material characteristics, uses, and mixing parameters.

The large size of silicon atoms (approximately 110 pm [10^-12 m], compared to 70 pm for carbon), as well as the Si-O-Si bond angle of 150° (compared to about 120° for C-C-C bonds in typical unbranched polymers) results in very little steric hindrance in silicones.² This allows for free rotation of the silicone molecule and flexibility at low temperatures (i.e., above T_g).^4,6 However, these structural properties also result in low mechanical strength.

Mixing Considerations

Silicones sealants are rarely pure silicone. Additives are incorporated to reduce cost; improve mechanical, thermal, and electrical properties; and provide other characteristics such as pigmentation, improved bonding to substrates, and UV resistance. Silicones are typically filled with 15-30% by weight silica. Although silica contains the same base material (silicon) as silicone, it exhibits differences in properties that improve the performance of the finished material. Silicas have strong polar surface characteristics; this is attributed to the hydroxyl groups occupying the surface of the silica particles.² This leads to silica bonding to itself, as opposed to the polymer molecules. While silica enables the reduced use of expensive polymers, the high loading can lead to challenges with mixing and dispersion processability of the uncured rubber compound.

Table 1 shows that the gas (and vapor) permeability of cured silicone is much greater than the representative organic polymers.⁴ The uncured and uncrosslinked product is infiltrated by gas and vapor more readily than the cured system. This leads to choosing different mixing and dispersing strategies than for polymers with lower gas permeability.

The building blocks for silicones are silanes, which are used extensively for their ability to bond inorganic and organic materials to one another, as well as for their water-scavenging properties. Silicones have some similar characteristics to the silanes used to form them, such as bonding ability with a variety of inorganic, organic, polar, and nonpolar substrates like metals, plastics, wood, and glass. Silicones are formed through reaction of the terminal hydroxy group reacting with a trifunctional curing molecule to produce a tetrafunctional silicone that is stable (uncured) in the absence of water vapor. Curing occurs through reaction with atmospheric moisture, whereby the water causes the silicone to hydrolyze and form hydroxyl end groups; these condense rapidly, crosslinking the polymer.²

Silicones have low thermal conductivity, which is advantageous in the finished product, as it is good for insulating. However, this can cause the product to detrimentally insulate itself during mixing. The silicone impedes the flow of heat out of the material. (As silicones for construction uses are quite viscous, the mixing process tends to impart much energy in the form of heat.) If a cooling jacket is placed around the mixing vessel, the area around the high-speed shaft impeller will be hotter and thinner; material near the jacket will be cooler and higher in viscosity. This temperature gradient can be minimized with the use of a helical sweep blade, which turns over the batch by way of vertical mixing as well as circulating the batch through horizontal mixing.

The “free volume”—essentially the ease with which a polymer chain can change conformation—is much lower in glassy polymers than in rubbery polymers. The free volume, and hence permeability, is related to the T_g through the Flory-Fox equation:^1,5

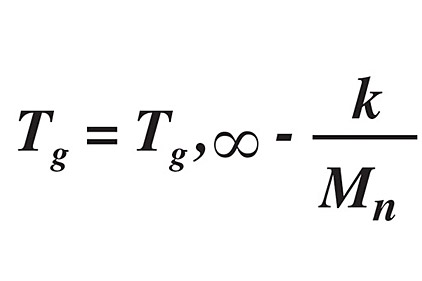
where T_g,∞ is the maximum glass transition temperature at a theoretical infinite polymeric molecular weight, k is a value related to the free volume, and M_n is the number average molecular weight of the polymer.

The T_g dictates the flow behavior of a polymer, to the extent that the T_g is a measure of free volume; in turn, free volume is a measure of permeability and diffusivity of the material. The viscosity change of a material as it is exposed to gases can be measured by the following equation:

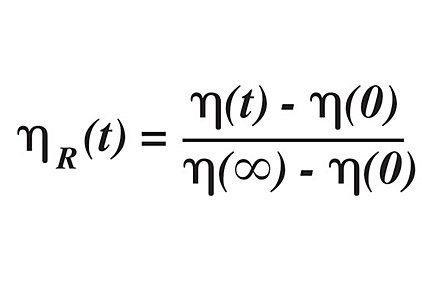
where the subscript R denotes relative viscosity at a specific time t (duration of exposure), 0 denotes the initial viscosity, and ∞ denotes the viscosity of the material at full gas saturation. From this equation, it can be seen that the viscosity decreases when air or other gaseous impurities are present.

As a polymer increases in molecular weight, and thus has an increased number of bonds, it can have a greater number of spatial conformations. Namely, each bond, relative to its neighbors, can be in the trans, gauche+, or gauche- states. For higher-molecular-weight polymers, this can lead to entanglement of the individual polymer chains, equating to a localized increase in viscosity.³ As the temperature is increased, there is more energy (i.e., entropy) in the system, thus a greater number of possible conformations; as temperature is decreased (or entanglement becomes quite large) the number of conformations is also decreased. At the T_g, the rate of change of conformation drops to zero, effectively “freezing” the polymer in its current state of conformation.⁶

Polydimethylsiloxanes, broadly known as silicones, exhibit viscoelastic properties when an external force is applied: at low mixing temperatures or short mix times they are rubber-like; at high mixing temperatures or prolonged mix times they are viscous liquids. The affect of this property is that the material does not respond evenly to loading and unloading, as can be seen in the hysteresis loop of a stress-strain curve for a viscoelastic material. The hysteresis loop effectively shows the amount of energy lost to the product in the form of heat, due to the internal friction of the material.^4,6 It is well-known that silicones heat as they are mixed; this is attributed not only to the viscosity, but to the viscoelastic properties of silicones.

A common, simplified model of viscoelasticity is the spring-dashpot model. The spring represents the elastic component (G’), while the dashpot represents the viscous component (G”). The ratio between these two components can be described by the equation of the loss tangent (tan d):^1,2,5

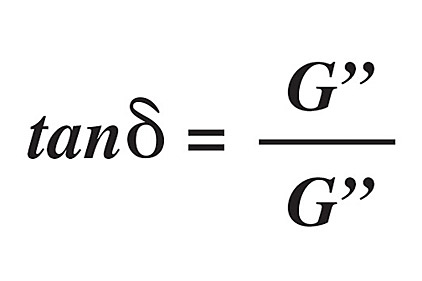
The loss tangent is referred to as a hysteresis, whereby a material’s behavior is influenced by past conditions. Graphically, this can be represented on a stress-strain diagram where loading and unloading exhibit different elastic moduli (see Figure 1). The shaded area between the two curves is the amount of energy lost; in the case of mixing of silicones, the energy is lost in the form of heat. It is important to note that hysteresis is the opposite of resilience; in other words, tan d is a viscous effect, as opposed to an elastic effect. This can be modeled another way through dynamic mechanical analysis by applying a sinusoidal stress while measuring the resultant strain. The following equation is powerful for measuring the viscoelastic properties, and determining T_g[2]:

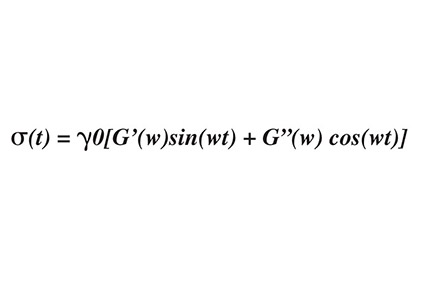
where G'(w) is the storage modulus, and G”(w) is the loss modulus. As stated above, G' is the elastic component, and is proportional to sin(wt), whereas G” is the viscous component, and is proportional to cos(wt). G'(w) and G"( w) (the storage and loss modulus, respectively) are functions of temperature and frequency; increasing the frequency has the same effect on the system as increasing the strain rate or reducing the temperature. The peak in the curve of tan d indicates a maximum rate of change in G'; this is also the T_g.²

Silicones, like all rubbers, exhibit thermoelasticity. The basis of thermoelasticity is molecular, but can be experienced in a quotidian way by stretching a rubber band. The rubber band heats up as it is elongated; this is a result of the energy of the system remaining fairly constant, while the work done to stretch the rubber band causes a decrease in the entropy of the polymeric network chains. The result that we experience is a warmer band, due to heat leaving the system.  This is akin to entropic elasticity, where applied forces are used to decrease the entropy of the polymer chains; and converse to energetic elasticity of materials such as metals, where internal energy changes are far greater than entropy changes. The “snap back” of a rubber band is a result of increasing the entropy and returning the system to a more favorable random state.

This is important to the mixing of silicones; silicones heat as they are mixed (elongated) just as the rubber band heats during elongation. The system wants to maintain a higher entropy state and “stay together”—this is one reason that silicones have a tendency to climb up the rotating disperser shaft as they are mixed. This effect, in a cured elastomer, is a result of vulcanization, the definition of which is “a process that increases retractile force and reduces the amount of permanent deformation remaining after removal of the deforming force ... vulcanization increases elasticity while decreasing plasticity.”²

Silicones are somewhat unique among sealants and adhesives, in that they have a much higher gas permeability than all systems that use carbon-chain backbone polymers. From a physics standpoint, this can be attributed to, and quantified by, Fick’s laws of diffusion, which relates the diffusive flux to concentration of diffusant. These equations were elaborated on by Einstein, becoming what is known as the Stokes-Einstein equation, which holds for the diffusion of spherical particles through liquids of low Reynolds number (i.e., in the laminar flow regime, viscous):⁵

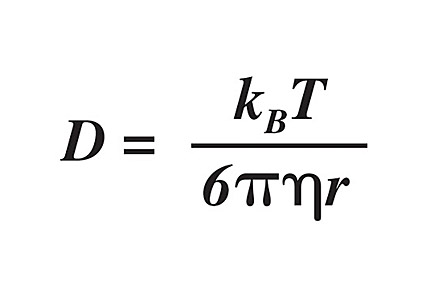
where D is the diffusion coefficient (diffusivity) with units of length squared per time, k_B is the Boltzmann’s constant, T is the temperature in Kelvin, h is the viscosity, and r is the radius of the spherical particle.

The gas permeability of silicone is generally on the order of 100 times greater than other sealants and materials based on butyl and nitrile rubber elastomers.⁴ Depending on the application, this can be beneficial; for example, medical devices made of silicone can be advantageous for their membrane-like nature, yet a dual-pane window sealed with silicone would not stand up to swings in temperature and humidity throughout the years, and would ultimately result in condensation between the panes of glass. The gas permeability is of importance during the mixing and dispersion of silicone materials. When mixing raw materials to disperse components, air will be incorporated into the mixture if there is no vacuum applied to the mix vessel.

The gas permeability of silicone can be used as a metric of the relative quantity of air, which can be anticipated to be entrapped in a mixed product. Table 1 shows that this quantity of air is substantial, and may lead to non-ideal product properties if not removed. One way to combat this is to use a mixing vessel equipped with vacuum. The air will expand as vacuum is increased, and the larger bubbles will escape easier than when they were small bubbles at atmospheric pressure.

Since silicones are 1-part (room temperature vulcanizing one-part, RTV-1) sealant systems (i.e., no solvents), vacuum can safely be pulled. When pulling vacuum, the low speed sweep and wiper blades are used to expedite the process of removing air and moisture. As the air expands, the product volume increases until bubbles are large enough to escape the mixture; a temporary increase in product volume necessitates adequate freeboard capacity of the vessel, so as to not damage vacuum lines and equipment.

Silicone sealants, such as those used in construction, tend to be thick, viscous materials, with viscosities of 50,000 centipoise (cP) or higher. By the definition of viscosity, products with higher viscosities are more resistant to flow when shear forces are applied, which accounts for silicone’s ability to stay in a vertical joint prior to curing without running. The finished material viscosity is clearly beneficial, yet during the mixing process the high viscosity can be challenging, requiring machine solutions to ensure that production plant efficiency and product requirements are met. The silicone sealants’ ability to withstand shear forces is evident in a number of ways in the production process, including the need for equipment to have greater horsepower and torque. The combined cohesive and adhesive forces within silicone sealant, coupled with the high viscosity (resistance to shear), results in the physical phenomena of the silicone “climbing” the rotating shaft. Silicones adhere both to themselves (cohesion) and to materials which they touch (adhesion), in this case vessel walls and shaft. As the shaft is rotating, the silicone tends to spread on it, a result of the wetting characteristics of the metal shaft-silicone physical properties interaction (Young’s equation). Silicone is highly adherent to itself as well, so the spreading up the shaft is not a thin layer, but thick; due to the cohesive forces of the material.

The tendency of silicones to climb up the shaft during mixing is due to the interplay of adhesive and cohesive forces. Should the product become too high up on the shaft, a “shaft slinger” can be used. To achieve this, a thin circular disc with a radius proportional to the mixing vessel is attached to the shaft above normal product mixing levels, such that any climbing will be mitigated. Once the silicone “climbs” to the height of the shaft slinger, it is forced outward (as it can no longer creep upward). Increasing distance from the shaft equates to greater rotational (centrifugal) forces, ultimately resulting in the silicone being slung off the shaft slinger and back down into the main body of the mix. The shaft slinger assists in transferring centripetal forces into centrifugal forces, thus keeping the material in the mixing zone. Figure 2 shows a dual-shaft mixer that would be suitable for mixing and dispersing viscous silicone products.*

Conclusion

Many factors must be taken into account when formulating a new silicone sealant. Careful consideration of the material properties and their interplay with the mixing process is a valuable component of end-product development. A well-formulated dispersion can only perform at its best when it is properly mixed.

For more information, contact the author by phone (323) 560-4723, ext. 216; fax (209) 354-4037; email sshira@myersmixer.com; or visit www.myersmixer.com.

1. Aklonis, John J., and MacKnight, William J., Introduction to Polymer Viscoelasticity, Hoboken, N.J., Wiley-Interscience, 2005.

2. Harper, Charles A., Handbook of Plastics Technologies: The Complete Guide to Properties and Performance, New York, McGraw-Hill, 2006.

3. Lin, Y.-H., Polymer Viscoelasticity: Basics, Molecular Theories, and Experiments, Singapore, World Scientific, 2003.

4. Mills, N.J., Plastics: Microstructure and Applications, Amsterdam, Elsevier Butterworth Heinemann, 2005.

5. Oswald, Patrick, Rheophysics: The Deformation and Flow of Matter, Cambridge, Cambridge UP, 2009.

 6. Saccomandi, Giuseppe, and Ogden, R.W., Mechanics and Thermomechanics of Rubberlike Solids, Wien, Springer, 2004.